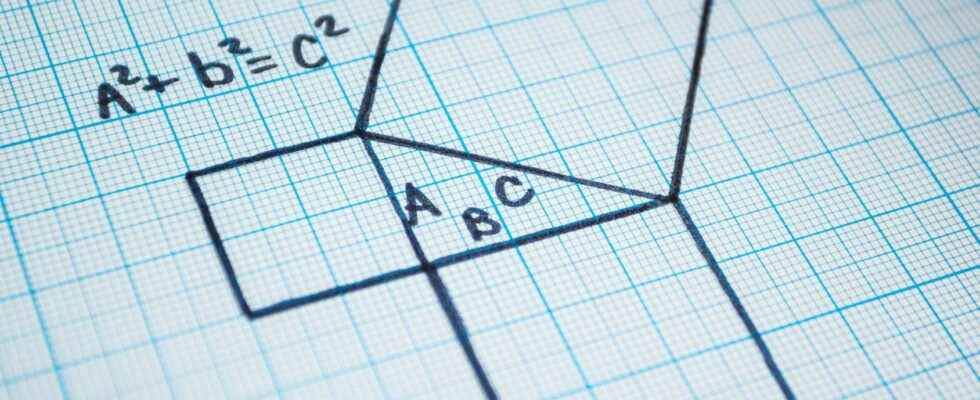
We attribute to Pythagoras, the theorem which affirms that, in a right-angled triangle, the square of the hypotenuse is equal to the sum of the squares of the two other sides… and vice versa: if this equality is true, the triangle is right-angled.
You will also be interested
We find the theorem of Pythagoras in all major civilizations. For example, in ancient China, it is called the Gougu theorem and there is an illustration of it in the Cabbage Peithe oldest Chinese mathematical treatise.
Indeed, in this figure appears a right triangle whose sides are 3, 4 and 5 and a proof that 32 + 42 = 52”.
What is the Egyptian triangle?
We call Egyptian triangle, the triangle of sides 3, 4 and 5. According to the theorem of Pythagoras, this triangle is right-angled.
In the Middle Ages, the contractors from cathedrals used a rope with thirteen knots (of length 12 = 5 + 4 + 3) to draw the right angles, using the reciprocal of the Pythagorean theorem since the equality 52 = 32 + 42 implies that the triangle of sides 3, 4 and 5 units is rectangle.
Since that time, the thirteen-knot rope carries the name of Egyptian triangle because the contractors traced its use back to ancient Egypt, which is in no way a proof that this is the case, because no Egyptian text accredits this hypothesis, which does not mean that it is false either.
An esoteric meaning?
The only ancient reference binder this Pythagorean triple to the Egyptians is found in the treaty ofIsis and Osiris (II, 56) of Plutarch (46 – 125). The Egyptians seem to have imagined the world in the form of the most beautiful of triangles; just as Plato, in his Republic, seems to have used it as a symbol of matrimonial union. This triangle has its vertical side composed of 3, the base of 4, the hypotenuse of 5 parts, and the square of this is equal to the sum of the squares of the other sides. The vertical side symbolizes the world, the base the female, and the hypotenuse the offspring of both. The least that can be said is that Plutarch did not see this triangle from a pragmatic but rather an esoteric point of view, and the same is true of Plato in The Republic (VIII, 546).
Interested in what you just read?
Subscribe to the newsletter Fun math : every week, Futura deals with a math question for the enjoyment of 7 to 77 year olds. All our newsletters